How to build a projection (Schlegel diagram) of a tesseract, and show a four-dimensional point inside it (II)

Some months ago I described in a post how to build a projection (Schlegel diagram) of a tesseract, and show a four-dimensional point inside it. Since then I have used it to review the symmetries of $(w,x,y,z,)$ four-dimensional tuples by observing the $3D$ projection of the cloud of points. 

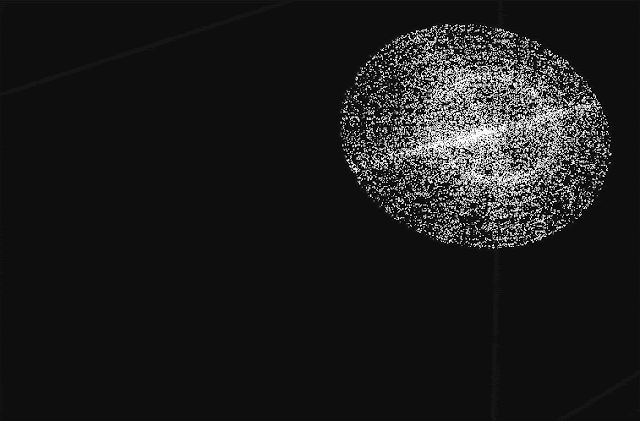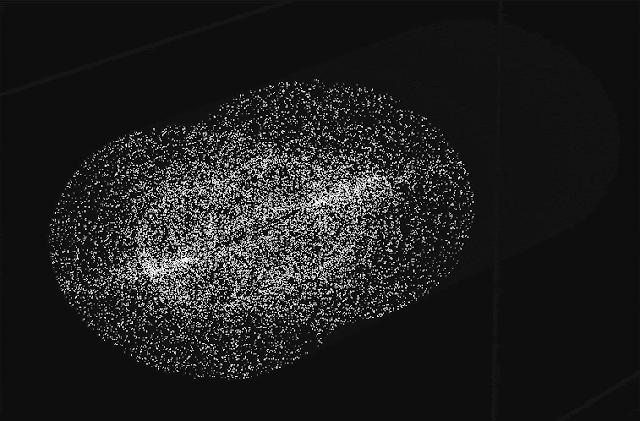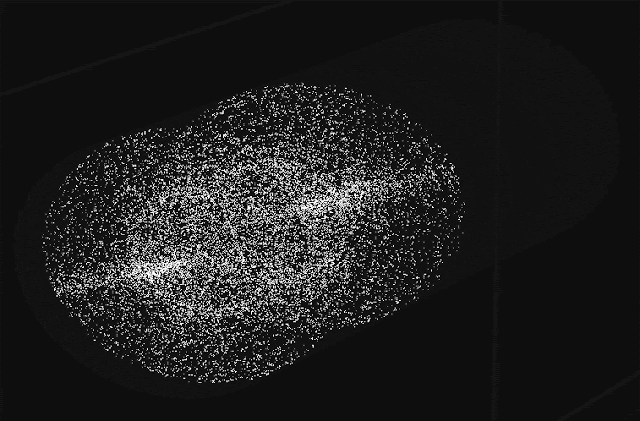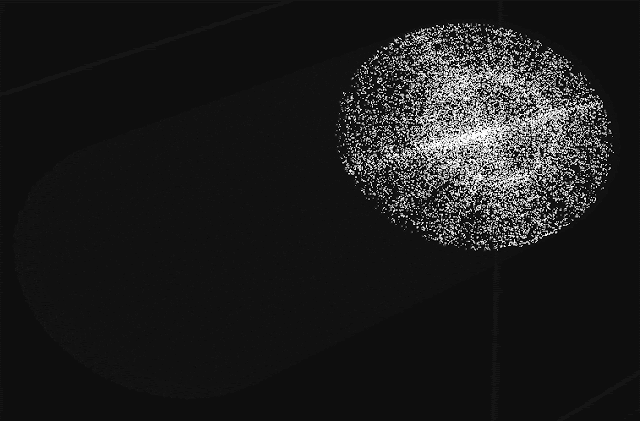
Recently I have been able to use it for a real problem of a MSE user. Here is the link to the question. I am really happy to have been useful to somebody else. This are two of the projections of the more than $4 \cdot 10^4$ four-dimensional cloud of points of the problem. They look gorgeous!

$(w, x, $Shadow of plane$(y,z))$:

 

$($Shadow of plane$(w,x), y, z)$: